We shall try and introduce coordinates which are appropriate to the problem. Since our choice of coordinates always lead to requirements on the metric functions, we must proceed carefully in order not to lose solutions by making the restrictions too strong.
Spherical symmetry evidently signifies that in the three dimensional space T= constant, all radial coordinates are equivalent and no perpendicular direction is singled out; in spherical polar coordinates we have [ for constant T ]
![]()
The most general ansatz for the line element in the full four- dimensional spacetime is therefore
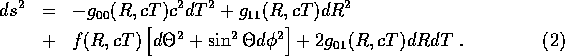
We can simplify this line element further using the coordinate transformation
![]()
so that
![]()
and
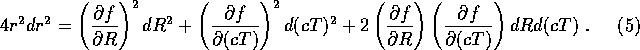
Using these results we can be bring the line element into the form
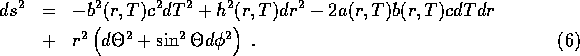
Notice that this already contains the usual two dimensional spherical surface element. A further transformation
![]()
eliminates the undesired non- diagonal terms. Thus we arrive at the Schwartzschild form of the line element of a spherically symmetric non- rotating body:
![]()